- Rocscience Slide 7.0
- Free Software Dips Roc Science Cracked
- Rocscience Slide 6.0
- Rocscience Software Download
DIPS adalah suatu program rancangan untuk menganalisis orientasi secara interaktif dengan mendasarkan data yang berhubungan dengan struktur geologi. Rocscience Phase2 v9.0.11 RS2 (Phase2 9.0) is a powerful 2D finite element program for soil and rock applications (RS2 = Rock and Soil 2-dimensional analysis program). RS2 can be used for a wide range of engineering projects and includes excavation design, slope stability, groundwater seepage, probabilistic analysis, consolidation, and dynamic.
Sample Results From Member DownloadsSlide Rocscience Crack. Convert Rocscience Slide 6.0 trail version to full software. Download Rocscience Slide v5.014 crack by Lz0 at our cracksguru database. Find lots of other cracks, serial numbers, keygens here. Rocscience slide v6.005 - download at 4shared. Rocscience slide v6.005 is hosted at free file sharing service 4shared.
Slide Rocscience Crack. Convert Rocscience Slide 6.0 trail version to full software. Download Rocscience Slide v5.014 crack by Lz0 at our cracksguru database. Find lots of other cracks, serial numbers, keygens here. Rocscience slide v6.005 - download at 4shared. Rocscience slide v6.005 is hosted at free file sharing service 4shared. Rocscience Softwares is a complete suite of software for geotechnical engineers. Since 1996, the company has been well positioned in the field of software development. The applications in this collection include a wide range of geotechnical and earth sciences tools that enable you to design and analyze 2D and 3D.
| Download Name | Date Added | Speed |
|---|---|---|
| Rocscience Slide | 08-Jun-2019 | 2,326 KB/s |
| Rocscience Slide (Jun/2019) | 08-Jun-2019 | 2,433 KB/s |
| Rocscience Slide Crack | 07-Jun-2019 | 2,202 KB/s |
| Rocscience Slide Full | 06-Jun-2019 | 2,735 KB/s |
| Rocscience Slide Password | 06-Jun-2019 | 2,967 KB/s |
| Rocscience.Slide_01.Jun.2019.rar | 01-Jun-2019 | 2,530 KB/s |
| Rocscience Slide ISO | 28-May-2019 | 2,222 KB/s |
Showing 7 download results of 7 for Rocscience Slide | ||
برچسب ها: download rocscience dips roc science roc science dips roc science slide roc science torrent rocscience rocscience dips rocscience dips crack rocscience dips download rocscience dips دانلود rocscience download rocscience examine2d download rocscience phase2 rocscience phase2 crack rocscience rocfall rocscience rockfall. Rocscience provides free, fully-functional trial copies of all our software. Please fill out the form below and a Rocscience representative will contact you soon to start your software trial.
Many downloads like Rocscience Slide may also include a crack, serial number, unlock code, cd key or keygen (key generator). If this is the case it is usually found in the full download archive itself.
To celebrate our launch we are offering unlimited full download access for FREE! This is a limited offer and will soon expire and revert back to the normal member price. We now have 288,035 downloads in the member section. Take the FileFixation tour now for more detailed information!
Design and Layout © 2019 FileFixation. All rights reserved.
Rocscience Slide 7.0
Slope stability analysis is performed to assess the safe design of a human-made or natural slopes (e.g. embankments, road cuts, open-pit mining, excavations, landfills etc.) and the equilibrium conditions.[1][2]Slope stability is the resistance of inclined surface to failure by sliding or collapsing.[3] The main objectives of slope stability analysis are finding endangered areas, investigation of potential failure mechanisms, determination of the slope sensitivity to different triggering mechanisms, designing of optimal slopes with regard to safety, reliability and economics, designing possible remedial measures, e.g. barriers and stabilization.[1][2]
Successful design of the slope requires geological information and site characteristics, e.g. properties of soil/rock mass, slope geometry, groundwater conditions, alternation of materials by faulting, joint or discontinuity systems, movements and tension in joints, earthquake activity etc.[4][5] The presence of water has a detrimental effect on slope stability. Water pressure acting in the pore spaces, fractures or other discontinuities in the materials that make up the pit slope will reduce the strength of those materials.[6]Choice of correct analysis technique depends on both site conditions and the potential mode of failure, with careful consideration being given to the varying strengths, weaknesses and limitations inherent in each methodology.[7]
Before the computer age stability analysis was performed graphically or by using a hand-held calculator. Today engineers have a lot of possibilities to use analysis software, ranges from simple limit equilibrium techniques through to computational limit analysis approaches (e.g. Finite element limit analysis, Discontinuity layout optimization) to complex and sophisticated numerical solutions (finite-/distinct-element codes).[1] The engineer must fully understand limitations of each technique. For example, limit equilibrium is most commonly used and simple solution method, but it can become inadequate if the slope fails by complex mechanisms (e.g. internal deformation and brittle fracture, progressive creep, liquefaction of weaker soil layers, etc.). In these cases more sophisticated numerical modelling techniques should be utilised. Also, even for very simple slopes, the results obtained with typical limit equilibrium methods currently in use (Bishop, Spencer, etc.) may differ considerably. In addition, the use of the risk assessment concept is increasing today. Risk assessment is concerned with both the consequence of slope failure and the probability of failure (both require an understanding of the failure mechanism).[8][9]
Within the last decade (2003) Slope Stability Radar has been developed to remotely scan a rock slope to monitor the spatial deformation of the face. Small movements of a rough wall can be detected with sub-millimeter accuracy by using interferometry techniques.
- 1Limit equilibrium analysis
- 1.1Analytical techniques: Method of slices
- 5Numerical methods of analysis
Limit equilibrium analysis[edit]
Conventional methods of slope stability analysis can be divided into three groups: kinematic analysis, limit equilibrium analysis, and rockfall simulators.[8]Most slope stability analysis computer programs are based on the limit equilibrium concept for a two- or three-dimensional model.[10][11] Two-dimensional sections are analyzed assuming plane strain conditions. Stability analyses of two-dimensional slope geometries using simple analytical approaches can provide important insights into the initial design and risk assessment of slopes.
Limit equilibrium methods investigate the equilibrium of a soil mass tending to slide down under the influence of gravity. Translational or rotational movement is considered on an assumed or known potential slip surface below the soil or rock mass.[12] In rock slope engineering, methods may be highly significant to simple block failure along distinct discontinuities.[8] All these methods are based on the comparison of forces, moments, or stresses resisting movement of the mass with those that can cause unstable motion (disturbing forces). The output of the analysis is a factor of safety, defined as the ratio of the shear strength (or, alternatively, an equivalent measure of shear resistance or capacity) to the shear stress (or other equivalent measure) required for equilibrium. If the value of factor of safety is less than 1.0, the slope is unstable.
All limit equilibrium methods assume that the shear strengths of the materials along the potential failure surface are governed by linear (Mohr-Coulomb) or non-linear relationships between shear strength and the normal stress on the failure surface.[12] The most commonly used variation is Terzaghi's theory of shear strength which states that
where is the shear strength of the interface, is the effective stress ( is the total stress normal to the interface and is the pore water pressure on the interface), is the effective friction angle, and is the effective cohesion.
The methods of slices is the most popular limit equilibrium technique. In this approach, the soil mass is discretized into vertical slices.[11][13] Several versions of the method are in use. These variations can produce different results (factor of safety) because of different assumptions and inter-slice boundary conditions.[12][14]
The location of the interface is typically unknown but can be found using numerical optimization methods.[15] For example, functional slope design considers the critical slip surface to be the location where that has the lowest value of factor of safety from a range of possible surfaces. A wide variety of slope stability software use the limit equilibrium concept with automatic critical slip surface determination.
Typical slope stability software can analyze the stability of generally layered soil slopes, embankments, earth cuts, and anchored sheeting structures. Earthquake effects, external loading, groundwater conditions, stabilization forces (i.e., anchors, geo-reinforcements etc.) can also be included.
Analytical techniques: Method of slices[edit]
Many slope stability analysis tools use various versions of the methods of slices such as Bishop simplified, Ordinary method of slices (Swedish circle method/Petterson/Fellenius), Spencer, Sarma etc. Sarma and Spencer are called rigorous methods because they satisfy all three conditions of equilibrium: force equilibrium in horizontal and vertical direction and moment equilibrium condition. Rigorous methods can provide more accurate results than non-rigorous methods. Bishop simplified or Fellenius are non-rigorous methods satisfying only some of the equilibrium conditions and making some simplifying assumptions.[13][14] Some of these approaches are discussed below.
Swedish Slip Circle Method of Analysis[edit]
The Swedish Slip Circle method assumes that the friction angle of the soil or rock is equal to zero, i.e., . In other words, when friction angle is considered to be zero, the effective stress term goes to zero, thus equating the shear strength to the cohesion parameter of the given soil. The Swedish slip circle method assumes a circular failure interface, and analyzes stress and strength parameters using circular geometry and statics. The moment caused by the internal driving forces of a slope is compared to the moment caused by forces resisting slope failure. If resisting forces are greater than driving forces, the slope is assumed stable.
Ordinary Method of Slices[edit]
In the method of slices, also called OMS or the Fellenius method, the sliding mass above the failure surface is divided into a number of slices. The forces acting on each slice are obtained by considering the mechanical (force and moment) equilibrium for the slices. Each slice is considered on its own and interactions between slices are neglected because the resultant forces are parallel to the base of each slice. However, Newton's third law is not satisfied by this method because, in general, the resultants on the left and right of a slice do not have the same magnitude and are not collinear.[16]
Rocscience Slide 7.0
This allows for a simple static equilibrium calculation, considering only soil weight, along with shear and normal stresses along the failure plane. Both the friction angle and cohesion can be considered for each slice. In the general case of the method of slices, the forces acting on a slice are shown in the figure below. The normal () and shear () forces between adjacent slices constrain each slice and make the problem statically indeterminate when they are included in the computation.
For the ordinary method of slices, the resultant vertical and horizontal forces are
where represents a linear factor that determines the increase in horizontal force with the depth of the slice. Solving for gives
Next, the method assumes that each slice can rotate about a center of rotation and that moment balance about this point is also needed for equilibrium. A balance of moments for all the slices taken together gives
where is the slice index, are the moment arms, and loads on the surface have been ignored. The moment equation can be used to solve for the shear forces at the interface after substituting the expression for the normal force:
Using Terzaghi's strength theory and converting the stresses into moments, we have
where is the pore pressure.The factor of safety is the ratio of the maximum moment from Terzaghi's theory to the estimated moment,
Modified Bishop’s Method of Analysis[edit]
The Modified Bishop’s method[17] is slightly different from the ordinary method of slices in that normal interaction forces between adjacent slices are assumed to be collinear and the resultant interslice shear force is zero. The approach was proposed by Alan W. Bishop of Imperial College. The constraint introduced by the normal forces between slices makes the problem statically indeterminate. As a result, iterative methods have to be used to solve for the factor of safety. The method has been shown to produce factor of safety values within a few percent of the 'correct' values.
The factor of safety for moment equilibrium in Bishop's method can be expressed as
where
where, as before, is the slice index, is the effective cohesion, is the effective internal angle of internal friction, is the width of each slice, is the weight of each slice, and is the water pressure at the base of each slice. An iterative method has to be used to solve for because the factor of safety appears both on the left and right hand sides of the equation.
Lorimer's method[edit]
Lorimer's Method is a technique for evaluating slope stability in cohesive soils. It differs from Bishop's Method in that it uses a clothoid slip surface in place of a circle. This mode of failure was determined experimentally to account for effects of particle cementation. The method was developed in the 1930s by Gerhardt Lorimer (Dec 20, 1894-Oct 19, 1961), a student of geotechnical pioneer Karl von Terzaghi.
Spencer’s Method[edit]
Spencer’s Method of analysis[18] requires a computer program capable of cyclic algorithms, but makes slope stability analysis easier. It is not as accurate as the Modified Bishop’s method, but is acceptably accurate in engineering practices.[19]
Sarma method[edit]
The Sarma method,[20] proposed by Sarada K. Sarma of Imperial College is a Limit equilibrium technique used to assess the stability of slopes under seismic conditions. It may also be used for static conditions if the value of the horizontal load is taken as zero. The method can analyse a wide range of slope failures as it may accommodate a multi-wedge failure mechanism and therefore it is not restricted to planar or circular failure surfaces. It may provide information about the factor of safety or about the critical acceleration required to cause collapse.
Comparisons[edit]
The assumptions made by a number of limit equilibrium methods are listed in the table below.[21]
| Method | Assumption |
|---|---|
| Ordinary method of cells | Interslice forces are neglected |
| Bishop's simplified/modified [17] | Resultant interslice forces are horizontal. There are no interslice shear forces. |
| Janbu's simplified[22] | Resultant interslice forces are horizontal. An empirical correction factor is used to account for interslice shear forces. |
| Janbu's generalized[22] | An assumed line of thrust is used to define the location of the interslice normal force. |
| Spencer [18] | The resultant interslice forces have constant slope throughout the sliding mass. |
| Chugh[23] | Same as Spencer's method but with a constant acceleration force on each slice. |
| Morgenstern-Price[24] | The direction of the resultant interslice forces is defined using an arbitrary function. The fractions of the function value needed for force and moment balance is computed. |
| Fredlund-Krahn (GLE) [16] | Similar to Morgenstern-Price. |
| Corps of Engineers [25] | The resultant interslice force is either parallel to the ground surface or equal to the average slope from the beginning to the end of the slip surface. |
| Lowe and Karafiath [26] | The direction of the resultant interslice force is equal to the average of the ground surface and the slope of the base of each slice. |
| Sarma [20] | The shear strength criterion is applied to the shears on the sides and bottom of each slice. The inclinations of the slice interfaces are varied until a critical criterion is met. |
The table below shows the statical equilibrium conditions satisfied by some of the popular limit equilibrium methods.[21]
| Method | Force balance (vertical) | Force balance (horizontal) | Moment balance |
|---|---|---|---|
| Ordinary MS | Yes | No | Yes |
| Bishop's simplified | Yes | No | Yes |
| Janbu's simplified | Yes | Yes | No |
| Janbu's generalized | Yes | Yes | Used to compute interslice shear forces |
| Spencer | Yes | Yes | Yes |
| Chugh | Yes | Yes | Yes |
| Morgenstern-Price | Yes | Yes | Yes |
| Fredlund-Krahn | Yes | Yes | Yes |
| Corps of Engineers | Yes | Yes | No |
| Lowe and Karafiath | Yes | Yes | No |
| Sarma | Yes | Yes | Yes |
Rock slope stability analysis[edit]
Rock slope stability analysis based on limit equilibrium techniques may consider following modes of failures:
- Planar failure -> case of rock mass sliding on a single surface (special case of general wedge type of failure); two-dimensional analysis may be used according to the concept of a block resisting on an inclined plane at limit equilibrium[27][28]
- Polygonal failure -> sliding of a nature rock usually takes place on polygonally-shaped surfaces; calculation is based on a certain assumptions (e.g. sliding on a polygonal surface which is composed from N parts is kinematically possible only in case of development at least (N - 1) internal shear surfaces; rock mass is divided into blocks by internal shear surfaces; blocks are considered to be rigid; no tensile strength is permitted etc.)[28]
- Wedge failure -> three-dimensional analysis enables modelling of the wedge sliding on two planes in a direction along the line of intersection[28][29]
- Toppling failure -> long thin rock columns formed by the steeply dipping discontinuities may rotate about a pivot point located at the lowest corner of the block; the sum of the moments causing toppling of a block (i.e. horizontal weight component of the block and the sum of the driving forces from adjacent blocks behind the block under consideration) is compared to the sum of the moments resisting toppling (i.e. vertical weight component of the block and the sum of the resisting forces from adjacent blocks in front of the block under consideration); toppling occur if driving moments exceed resisting moments[30][31]
Limit equilibrium analysis software[edit]
- SLIDE[32] provides 2D stability calculations in rocks or soils using these rigorous analysis methods: Spencer,Sarma and Morgenstern-Price/General limit equilibrium; and non-rigorous methods: Bishop simplified, Corps of Engineers, Janbu simplified/corrected, Lowe-Karafiath and Ordinary/Fellenius. Searching of the critical slip surface is realized with the help of a grid or as a slope search in user-defined area. Program includes also probabilistic analysis using Monte Carlo or Latin Hypercube simulation techniques where any input parameter can be defined as a random variable. Probabilistic analysis determine the probability of failure and reliability index, which gives better representation of the level of safety. Back analysis serves for calculation of a reinforcement load with a given required factor of safety. Program enables finite element groundwater seepage analysis.[32]
- SLOPE/W[33] is formulated in terms of moment and force equilibrium factor of safety equations. Limit equilibrium methods include Morgenstern-Price, General limit equilibrium, Spencer, Bishop, Ordinary, Janbu etc. This program allows integration with other applications. For example, finite element computed stresses from SIGMA/W[34] or QUAKE/W[35] can be used to calculate a stability factor by computing total shear resistance and mobilized shear stress along the entire slip surface. Then a local stability factor for each slice is obtained. Using a Monte Carlo approach, program computes the probability of failure in addition to the conventional factor of safety.[33]STABL WV[36] is a limit equilibrium-based, Windows software based on the stabl family of algorithms. It allows analysis using Bishop's, Spencer's and Janbu's method. Regular slopes as well as slopes with various types of inclusions may be analyzed.
- HYDRUS[37] add-on modules can check the stability of embankments, dams, earth cuts and anchored sheeting structures with the influence of the water. The values of the pore pressure in transport domain are imported automatically for the selected time to Stability module. The analysis can be repeated for all time shots of the water movement simulated by basic program. The common method of slices (the Bishop, Fellenius/Petterson, Morgenstern-Price or the Spencer) can be set as well as the different type of Geo-reinforcement or Earthquake effects.
- SVSlope[38] is formulated in terms of moment and force equilibrium factor of safety equations. Limit equilibrium methods include Morgenstern-Price, General limit equilibrium, Spencer, Bishop, Ordinary, Kulhawy and others This program allows integration with other applications in the geotechnical software suite. For example, finite element computed stresses from SVSolid[39] or pore-water pressures from SVFlux[40] can be used to calculate the factor of safety by computing total shear resistance and mobilized shear stress along the entire slip surface. The software also utilizes Monte Carlo, Latin Hypercube, and the APEM probabilistic approaches. Spatial variability through random fields computations may also be included in the analysis.
- Scoops3D[41]evaluates slope stability throughout a digital landscape represented by a digital elevation model (DEM). The program uses a three-dimensional (3D) method of columns limit-equilibrium analysis (Bishop's simplified or Ordinary methods) to assess the stability of many potential rotational landslides (typically millions) within a user-defined size range. It provides the least-stable potential landslide for each DEM cell in the landscape, as well the associated volumes and (or) areas. It can incorporate full 3D distributions of material properties and pore pressures.
- dotSlope[42] provides limit equilibrium analyses through the methods of Fellenius, Bishop simplified, Janbu simplified/corrected, Corps of Engineers, Lowe & Karafiath, Spencer, GLE/Morgenstern & Price. The slope can have multiple soils, impenetrable layers, cuts and embankments, multiple groundwater conditions, ponded water, dry and water filled tension cracks, soil reinforcements (anchors, nails, piles and geo-synthetics). Slip surfaces can be defined through six surface generators in order to find the critical case. dotSlope[42] can run deterministic analyses, surface optimization, sensitivity analyses along with probabilistic analyses using the Monte Carlo method. The program runs on Microsoft Windows, Mac OS X and Android.[43]
- GALENA[44] - includes stability analysis, back analysis, and probability analysis, using the Bishop, Spencer-Wright and Sarma methods.[44]
- GSLOPE[45] - provides limit equilibrium slope stability analysis of existing natural slopes, unreinforced man-made slopes, or slopes with soil reinforcement, using Bishop’s Modified method and Janbu’s Simplified method applied to circular, composite or non-circular surfaces.[45]
- CLARA-W[46] - three-dimensional slope stability program includes calculation with the help of these methods: Bishop simplified, Janbu simplified, Spencer and Morgenstern-Price. Problem configurations can involve rotational or non-rotational sliding surfaces, ellipsoids, wedges, compound surfaces, fully specified surfaces and searches.[46]
- TSLOPE3[47] - two- or three-dimensional analyses of soil and rock slopes using Spencer method.[47]
- A program specific for rock slope analysis isAutoBlock.[48] It overcome two problems inherently complicating the analysis in engineering practice: firstly determining volumes and magnitudes of sliding areas of potentially unstable blocks based on the real topography, and secondly finding the critical blocks which are formed by an intersection of various discontinuities. It allows importing arbitrarily complex terrain surfaces which have been digitized beforehand using a topographic map. These surfaces are then extruded to a 3D solid which may be intersected by various sets of discontinuities. By combining all possible locations of all discontinuities potentially unstable blocks are determined. For each block, the factor of safety against sliding is computed using the limit equilibrium method. AutoBlock is an add-on to the popular program 'AutoCAD' and exploits its possibilities and its power (e.g. for 3D-visualizations).
Limit analysis[edit]
A more rigorous approach to slope stability analysis is limit analysis. Unlike limit equilibrium analysis which makes ad-hoc though often reasonable assumptions, limit analysis is based on rigorous plasticity theory. This enables, among other things, the computation of upper and lower bounds on the true factor of safety.
Programs based on limit analysis include:
- OptumG2 (2014-) General purpose software for geotechnical applications including slope stability.
- LimitState:GEO (2008-) General purpose geotechnical software application based on Discontinuity layout optimization for plane strain problems including slope stability.
- GEO5 Slope Stability (1989-) Program is used to perform slope stability analysis of embankments, earth cuts, anchored retaining structures and MSE walls.
Stereographic and kinematic analysis[edit]
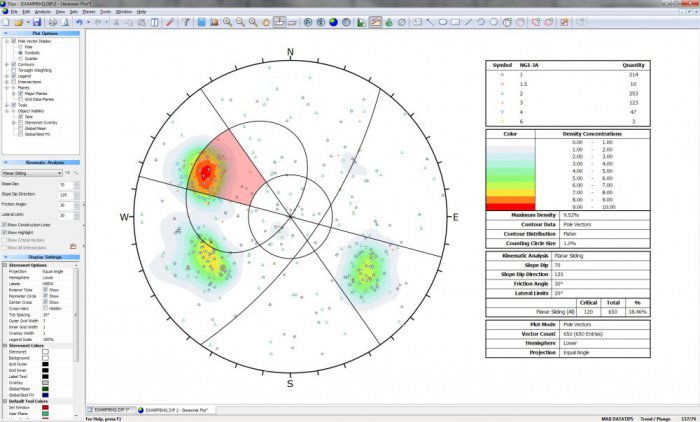
Kinematic analysis examines which modes of failure can possibly occur in the rock mass. Analysis requires the detailed evaluation of rock mass structure and the geometry of existing discontinuities contributing to block instability.[49][50]Stereographic representation (stereonets) of the planes and lines is used.[51] Stereonets are useful for analyzing discontinuous rock blocks.[52] Program DIPS[53] allows for visualization structural data using stereonets, determination of the kinematic feasibility of rock mass and statistical analysis of the discontinuity properties.[49][53] Free download nada sms lucu terbaru.
Rockfall simulators[edit]
Rock slope stability analysis may design protective measures near or around structures endangered by the falling blocks. Rockfall simulators determine travel paths and trajectories of unstable blocks separated from a rock slope face. Analytical solution method described by Hungr & Evans[54] assumes rock block as a point with mass and velocity moving on a ballistic trajectory with regard to potential contact with slope surface. Calculation requires two restitution coefficients that depend on fragment shape, slope surface roughness, momentum and deformational properties and on the chance of certain conditions in a given impact.[55]
Program ROCFALL[56] provides a statistical analysis of trajectory of falling blocks. Method rely on velocity changes as a rock blocks roll, slide or bounce on various materials. Energy, velocity, bounce height and location of rock endpoints are determined and may be analyzed statistically. The program can assist in determining remedial measures by computing kinetic energy and location of impact on a barrier. This can help determine the capacity, size and location of barriers.[56]
Numerical methods of analysis[edit]

Numerical modelling techniques provide an approximate solution to problems which otherwise cannot be solved by conventional methods, e.g. complex geometry, material anisotropy, non-linear behaviour, in situ stresses. Numerical analysis allows for material deformation and failure, modelling of pore pressures, creep deformation, dynamic loading, assessing effects of parameter variations etc. However, numerical modelling is restricted by some limitations. For example, input parameters are not usually measured and availability of these data is generally poor. Analysis must be executed by well trained user with good modelling practise. User also should be aware of boundary effects, meshing errors, hardware memory and time restrictions. Numerical methods used for slope stability analysis can be divided into three main groups: continuum, discontinuum and hybrid modelling.[57]
Continuum modelling[edit]
Modelling of the continuum is suitable for the analysis of soil slopes, massive intact rock or heavily jointed rock masses. This approach includes the finite-difference and finite element methods that discretize the whole mass to finite number of elements with the help of generated mesh (Fig. 3). In finite-difference method (FDM) differential equilibrium equations (i.e. strain-displacement and stress-strain relations) are solved. finite element method (FEM) uses the approximations to the connectivity of elements, continuity of displacements and stresses between elements. Most of numerical codes allows modelling of discrete fractures, e.g. bedding planes, faults. Several constitutive models are usually available, e.g. elasticity, elasto-plasticity, strain-softening, elasto-viscoplasticity etc.[57]
Discontinuum modelling[edit]
Discontinuum approach is useful for rock slopes controlled by discontinuity behaviour. Rock mass is considered as an aggregation of distinct, interacting blocks subjected to external loads and assumed to undergo motion with time. This methodology is collectively called the discrete-element method (DEM). Discontinuum modelling allows for sliding between the blocks or particles. The DEM is based on solution of dynamic equation of equilibrium for each block repeatedly until the boundary conditions and laws of contact and motion are satisfied. Discontinuum modelling belongs to the most commonly applied numerical approach to rock slope analysis and following variations of the DEM exist:[57]
- distinct-element method
- discontinuous deformation analysis (DDA)
- particle flow codes
The distinct-element approach describes mechanical behaviour of both, the discontinuities and the solid material. This methodology is based on a force-displacement law (specifying the interaction between the deformable rock blocks) and a law of motion (determining displacements caused in the blocks by out-of-balance forces). Joints are treated as [boundary conditions. Deformable blocks are discretized into internal constant-strain elements.[57]
Discontinuum program UDEC[58] (Universal distinct element code) is suitable for high jointed rock slopes subjected to static or dynamic loading. Two-dimensional analysis of translational failure mechanism allows for simulating large displacements, modelling deformation or material yielding.[58] Three-dimensional discontinuum code 3DEC[59] contains modelling of multiple intersecting discontinuities and therefore it is suitable for analysis of wedge instabilities or influence of rock support (e.g. rockbolts, cables).[57]
In discontinuous deformation analysis (DDA) displacements are unknowns and equilibrium equations are then solved analogous to finite element method. Each unit of finite element type mesh represents an isolated block bounded by discontinuities. Advantage of this methodology is possibility to model large deformations, rigid body movements, coupling or failure states between rock blocks.[57]
Discontinuous rock mass can be modelled with the help of distinct-element methodology in the form of particle flow code, e.g.program PFC2D/3D.[60][61] Spherical particles interact through frictional sliding contacts. Simulation of joint bounded blocks may be realized through specified bond strengths. Law of motion is repeatedly applied to each particle and force-displacement law to each contact. Particle flow methodology enables modelling of granular flow, fracture of intact rock, transitional block movements, dynamic response to blasting or seismicity, deformation between particles caused by shear or tensile forces. These codes also allow to model subsequent failure processes of rock slope, e.g. simulation of rock[57]

Hybrid/coupled modelling[edit]
Hybrid codes involve the coupling of various methodologies to maximize their key advantages, e.g. limit equilibrium analysis combined with finite element groundwater flow and stress analysis adopted in the SVOFFICE[62] or GEO-STUDIO[63] suites of software; coupled particle flow and finite-difference analyses used in PF3D[61] and FLAC3D.[64] Hybrid techniques allows investigation of piping slope failures and the influence of high groundwater pressures on the failure of weak rock slope. Coupled finite-/distinct-element codes, e.g. ELFEN,[65] provide for the modelling of both intact rock behaviour and the development and behaviour of fractures.[57]
Rock mass classification[edit]
Various rock mass classification systems exist for the design of slopes and to assess the stability of slopes. The systems are based on empirical relations between rock mass parameters and various slope parameters such as height and slope dip.
The Q-slope method for rock slope engineering and rock mass classification developed by Barton and Bar[66] expresses the quality of the rock mass for assessing slope stability using the Q-slope value, from which long-term stable, reinforcement-free slope angles can be derived.
See also[edit]
References[edit]
- ^ abcEberhardt 2003, p. 4
- ^ abAbramson 2002, p. 2
- ^Kliche 1999, p. 2
- ^USArmyCorps 2003, pp. 1–2
- ^Abramson 2002, p. 1
- ^Beale, Geoff; Read, John, eds. (2014). Guidelines for Evaluating Water in Pit Slope Stability. CSIRO Publishing. ISBN9780643108356.
- ^Stead 2001, p. 615
- ^ abcEberhardt 2003, p. 6
- ^Cardenas, IC (2019). 'On the use of Bayesian networks as a meta-modelling approach to analyse uncertainties in slope stability analysis'. Georisk: Assessment and Management of Risk for Engineered Systems and Geohazards. 13 (1): 53–65. doi:10.1080/17499518.2018.1498524.
- ^Abramson 2002, p. 329
- ^ abAbramson 2002, p. 363
- ^ abcUSArmyCorps 2003, p. 2
- ^ abZhu 2003, pp. 377–395
- ^ abAbramson 2002, pp. 363–367
- ^USArmyCorps 2003, p. 5
- ^ abFredlund, DG; Krahn, J (1977), 'Comparison of slope stability methods of analysis', Canadian Geotechnical Journal, 14 (3): 429–439
- ^ abBishop, A. W. (1955). 'The use of the Slip Circle in the Stability Analysis of Slopes'. Géotechnique. 5: 7. doi:10.1680/geot.1955.5.1.7.
- ^ abSpencer, E. (1967). 'A Method of analysis of the Stability of Embankments Assuming Parallel Inter-Slice Forces'. Géotechnique. 17: 11. doi:10.1680/geot.1967.17.1.11.
- ^Coduto, Donald; et al. (2011). Geotechnical Engineering Principles and Practices. New Jersey: Pearson Higher Education. ISBN9780132368681.
- ^ abSarma, S. K. (1975). 'Seismic stability of earth dams and embankments'. Géotechnique. 25 (4): 743. doi:10.1680/geot.1975.25.4.743.
- ^ abFredlund, D.G. (1984), 'Analytical methods for slope stability analysis', Proceedings of the Fourth International Symposium on Landslides, State-of-the-Art: 229–250
- ^ abJanbu, Nilmar (1973), RC Hirschfeld and SJ Poulos (eds.), 'Slope stability computations', In Embankment-dam Engineering, Jon Wiley and Sons Inc., NY: 40PCS1 maint: Uses editors parameter (link)
- ^Chugh, Ashok K (1982), 'Slope stability analysis for earthquakes', International Journal for Numerical and Analytical Methods in Geomechanics, 6 (3): 307–322., Bibcode:1982IJNAM..6.307C, doi:10.1002/nag.1610060304
- ^Morgenstern, N. R.; Price, V. Eo (1965), 'The analysis of the stability of general slip surfaces', Geotechnique, 15 (1): 79–93
- ^'Slope Stability'(PDF). US Army Corps of Engineers. Retrieved 15 April 2015.
- ^Lowe, John; Karafiath, Leslie (1960), 'Stability of earth dams upon drawdown', In Proc. 1st. Pan American Conference on Soil Mechanics and Foundation Engineering, México, 2: 537–552
- ^Kliche 1999, pp. 125–137
- ^ abcKovari 1978, pp. 103–124
- ^Kliche 1999, pp. 153–169
- ^Kliche 1999, p. 15
- ^Kliche 1999, pp. 139–152
- ^ ab'SLIDE – 2D Limit Equilibrium Slope Stability Analysis', Rocscience.com, Toronto, Canada: Rocscience, retrieved 20 July 2009
- ^ ab'SLOPE/W – Slope Stability Analysis', Geo-Slope.com, Calgary, Canada: Geo-Slope International, retrieved 20 July 2009
- ^'SIGMA/W – Stress-deformation Analysis', Geo-Slope.com, Calgary, Canada: Geo-Slope International, retrieved 21 July 2009
- ^'QUAKE/W – Dynamic Earthquake Analysis', Geo-Slope.com, Calgary, Canada: Geo-Slope International, retrieved 21 July 2009
- ^'STABL WV – Slope Stability Analysis Software', GeotechnicalSoftware.biz, Miami, FL: TerraWiz, LLC
- ^'HYDRUS – Slope Stability Module', PC-Progress.com, Prague, CR: PC-Progress s.r.o.
- ^'SVSlope – Slope Stability Analysis', SoilVision.com, Saskatoon, Canada: SoilVision Systems Ltd., retrieved 20 July 2010
- ^'SVSolid – Stress-deformation Analysis', SVSolid.com, Saskatoon, Canada: SoilVision Systems Ltd., retrieved 24 July 2010
- ^'SVFlux – Groundwater Seepage Analysis', SoilVision.com, Saskatoon, Canada: SoilVision Systems Ltd., retrieved 24 July 2010
- ^Reid, Mark E.; Christian, Sarah B.; Brien, Dianne L.; Henderson, Scott T. (2015). 'Scoops3D: software to analyze 3D slope stability throughout a digital landscape'. Techniques and Methods. doi:10.3133/tm14a1. ISSN2328-7055.
- ^ ab'dotSlope – The Modern Slope Stability Analysis', DotSlope.com, Italy: Nebulare, retrieved 16 March 2015
- ^'dotSlope for Android', Play.Google.com/store, Google Play Store, retrieved 16 March 2015
- ^ ab'GALENA – Slope Software System', SciSoftware.com, Utah, USA: Scientific Software Group, retrieved 20 July 2009
- ^ ab'GSLOPE – Limit Equilibrium Slope Stability Analysis for Reinforced Slopes', MitreSoftware.com, Edmonton, Canada: Mitre Software Corporation, retrieved 20 July 2009
- ^ ab'CLARA-W – 2D and 3D Slope Stability Analysis', Clara-w.com/, West Vancouver, Canada: O. Hungr Geotechnical Research, retrieved 21 July 2009
- ^ ab'TSLOPE3 – 2D and 3D Analyses of Soil and Rock Slopes', TAGASoft.com, California, USA: TAGA Engineering Software, retrieved 21 July 2009
- ^Computer Program AutoBlock for Analyzing the Stability of Foundations and Slopes in Rock based on a Digital Terrain Model, Zurich, Switzerland: ETH
- ^ abEberhardt 2003, p. 7
- ^Kliche 1999, p. 111
- ^Kliche 1999, pp. 111–123
- ^Kliche 1999, pp. 43–65
- ^ ab'DIPS – Graphical and Statistical Analysis of Orientation Data', Rocscience.com, Toronto, Canada: Rocscience, retrieved 21 July 2009
- ^Hungr 1988, pp. 685–690
- ^Eberhardt 2003, pp. 15–17
- ^ ab'ROCFALL – Statistical Analysis of Rockfalls', Rocscience.com, Toronto, Canada: Rocscience, retrieved 21 July 2009
- ^ abcdefghEberhardt 2003, pp. 17–38
- ^ ab'UDEC - Universal Distinct Element Code', Itascacg.com, Minneapolis, USA: Itasca, retrieved 27 July 2009
- ^'3DEC - Three Dimensional Distinct Element Code', Itascacg.com, Minneapolis, USA: Itasca, retrieved 27 July 2009
- ^'PFC2D - Particle Flow Code in Two Dimensions', Itascacg.com, Minneapolis, USA: Itasca, retrieved 27 July 2009
- ^ ab'PFC3D - Particle Flow Code in Three Dimensions', Itascacg.com, Minneapolis, USA: Itasca, retrieved 27 July 2009
- ^'SVOffice - Next generation geotechnical software', SoilVision.com, Saskatoon, Canada: SoilVision Systems Ltd, retrieved 1 August 2009
- ^'GEO-STUDIO - Suite of software for geotechnical modelling', Geo-Slope.com, Calgary, Canada: Geo-Slope International, retrieved 1 August 2009
- ^'FLAC3D - Fast Langrangian Analysis of Continua in Three Dimensions', Itascacg.com, Minneapolis, USA: Itasca, retrieved 2 August 2009
- ^'ELFEN - 2D/3D numerical modelling package', Rockfield.co.uk, West Glamorgan, U.K.: Rockfield Software, retrieved 2 August 2009
- ^Bar, N.; Barton, N.R. (2017). 'The Q-slope Method for Rock Slope Engineering'. Rock Mechanics & Rock Engineering, Vol 50, Springer, Vienna, https://doi.org/10.1007/s00603-017-1305-0.
Free Software Dips Roc Science Cracked
Bibliography[edit]
- Kliche, Charles A. (1999), Rock Slope Stability, Colorado, USA: Society for Mining, Metallurgy, and Exploration, ISBN0-87335-171-1
- Eberhardt, Erik (2003), Rock Slope Stability Analysis - Utilization of Advanced Numerical Techniques(PDF), Vancouver, Canada: Earth and Ocean Sciences, University of British Columbia
- US Army Corps of Engineers (2003), Engineering and Design - Slope Stability(PDF), Washington DC, USA: US Army Corps of Engineers
- Stead, Doug; Eberhardt, E.; Coggan, J.; Benko, B. (2001), M. Kühne; H.H. Einstein; E. Krauter; H. Klapperich; R. Pöttler (eds.), Advanced numerical techniques in rock slope stability analysis - Applications and limitations(PDF), Davos, Switzerland: Verlag Glückauf GmbH, pp. 615–624
- Abramson, Lee W.; Lee, Thomas S.; Sharma, Sunil; Boyce, Glenn M. (2002), Slope Stability and Stabilization Methods (2nd ed.), New York, USA: John Wiley & Sons, ISBN0-471-38493-3
- Zhu, D.Y.; Lee, C.F.; Jiang, H.D. (2003), 'Generalised framework of limit equilibrium methods for slope stability analysis', Geotechnique, Telford, London, Great Britain, 53 (4): 377–395, doi:10.1680/geot.2003.53.4.377, ISSN0016-8505
- Kovári, Kalman; Fritz, P. (1978), Slope Stability with Plane, Wedge and Polygonal Sliding Surfaces, Rio de Janeiro, Brazil, pp. 103–124
- Yang, Xiao-Li; Li, L.; Yin, J.H. (2004), 'Stability analysis of rock slopes with a modified Hoek-Brown failure criterion', International Journal for Numerical and Analytical Methods in Geomechanics, Chichester, Great Britain: John Wiley & Sons, 28 (2): 181–190, Bibcode:2004IJNAM.28.181Y, doi:10.1002/nag.330, ISSN0363-9061
- Barton, N.R.; Bandis, S.C. (1990), Barton, Nick (ed.), Review of predictive capabilities of JRC-JCS model in engineering practice, Rotterdam: Balkema, pp. 603–610, ISBN978-90-6191-109-8
- Hungr, O.; Evans, S.G. (1988), Bonnard, C. (ed.), Engineering evaluation of fragmental rockfall hazards, Rotterdam: Balkema, pp. 685–690
External links[edit]
Rocscience Slide 6.0
- Geotechnical Software at Curlie